Bell tuning in ancient China: a six-tone scale in a 12-tone system based on fifths and thirds
Martin Braun
Neuroscience of Music, S-671 95 Klässbol, Sweden
Date of publication: June 16, 2003
Abstract
A unitary ensemble of 65 bells, with 130 discrete strike tones, was excavated
in a fully preserved state 1978 in the Chinese province of Hubei from
the tomb of the Marquis Yi of Zeng from 433 B.C. The ensemble's tuning
system could now for the first time be determined by applying exclusively
empirical methods. Only pitch data, hanging order, and tone names on the
bells were considered. The results show (1) a norm tone of F4 ~ 345 Hz
(ca. F4-20 Cent, re modern A4 = 440 Hz), (2) a six-tone standard scale
of D-E-F-G-A-C with F#, G#, A#, B, C#, and D# as accidentals, and (3)
a third-oriented tuning with equally tempered fifths (~696 Cent) in the
series CGDAE.

Sound sample (mp3, 501 KB, 31 sec) from a test performance with the original bells, well demonstrating single-bell sounds but not orchestral ones.
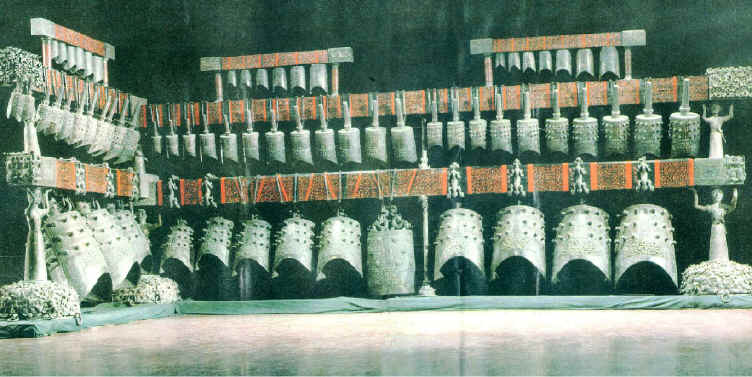
The 65 bronze bells from the tomb of Marquis Yi of Zeng (433 B.C.) are considered as one of the most fortunate discoveries in archeology. Due to favorable conditions of tomb preparation, soil, and soon natural water filling, the bronze of the bells survived fully intact. The bells sound as they did 2,436 years ago, and - as yet - better than any replicas that have been made in our days.
Two tones per bell
Two-tone bells were common in China between 1200 and 200 B.C. After that
the advanced know-how to cast them was lost, and it could not yet be fully
recovered in our days. The bells have an eye-shaped cross-section and
vibrate in one of two modes, depending on where they are struck. A strike
in the middle of the front makes frontside and backside vibrate as whole
units and produces the lower tone sui. A strike between the middle
of the front and a side edge makes frontside and backside vibrate as two
units each and produces the higher tone gu. If struck correctly,
both tones are fully independent, each with its own fundamental and harmonics.This
is shown in a spectral analysis, presented together
with the underlying sound samples, of the two tones of one of the
original bells. Quality and timbre of the two tones are fully compatible,
and they are both marked by tone-name inscriptions on the bells. Thus,
both were intended to be used in musical practice. Of the 33 bells in
the melody section of the Zeng ensemble, 21 have a sui-gu interval of
a minor third (mean 312.4 Cent, SD 13.8), 10 have one of a major third
(mean 403.1 Cent, SD 18.1), and two have apparently mistuned ones with
244 and 342 Cent (details below).
Tone name system
The tone names inscribed on the bells very clearly reflect a theory of
scale generation by both fifths and thirds. It must be examined, however,
if the actual tuning practice agrees with the theory. The results show
that it does not. The tone name system obviously had lost its theoretical
background for the bell casters (details below). But it is a very interesting
name system, because it shows an awareness of the thirds in the concept
of scale construction, something that developed in Europe ca. 2000 years
later.
gong jue, E
gong, C
gong zeng, G#
zhi jue, B
zhi, G
zhi zeng, D#
shang jue, F#
shang, D
shang zeng, A#
yu jue, C#
yu [yü], A
yu zeng, F
Hanging order and performance options
As shown in the pictures above, all bells are united in one L-shaped suspension
system. The 13 bass bells in the lower tier were possibly used for punctuation,
like the gongs in gamelan music. The 19 small bells in the upper tier
are tuned in deviating modes, and less accurately than the melody bells.
They may have been used for special effects. The bells of the lower and
upper tiers roughly match the 12-tone system of the 33 melody bells in
the middle tier. But due to tuning deviations they can not have participated
in a "tutti" sound, as performed by string instruments in a
European orchestra, or by sarons and bonangs in a Gamelan orchestra.
Scale D-E-F-G-A-C occurs eight times in melody bells
Analysis of the measured tone data shows that the 33 melody bells in the
middle tier repeat the six-tone scale D-E-F-G-A-C eight times. In five
of the eight cases, the scale exists as a subsection of just three adjacent
bells that are part of one of the three sections of the middle tier with
11, 12, and 10 bells, respectively (see pictures). Each of the five three-bell
groups provides the six tones DEFGAC due to the two-tone character of
one bell: first bell D-F, second bell E-G, and third bell A-C. Because
the bell sounds have short decay times - another accomplishment of the
sophisticated bell shape - the availability of a complete octave scale
in three adjacent bell allows very fast playing. The apparent order of
octave repetition reveals a subdivision of the three bell rows of the
middle tier into 9 groups of 3-5 bells each, as shown in the table below.
One can assume that in some performance types there was a player for each
of these 9 subgroups. That way it was possible to play eight-fold "tutti"
melodies, very similarly as today with saron instruments in a Gamelan
orchestra.
Set III - right
Interestingly, the DEFGAC scale uses the same tones as the famous hexachord of Guido of Arezzo from the early 11th century A.C., which was the foundation of European music notation. The differences are that Guido's hexachord was (1) a purely relative pitch system with an interval structure of CDEFGA, and (2) unrelated to any norm tone, an invention that had little relevance in Europe before the 19th century.
Scale D-E-F-G-A-C as key to tuning system
To my knowledge, the musical order of the 33 melody bells of the Zeng
ensemble has never been published before. This shortcoming may have prevented
a complete analysis of the bell's tuning system. As will be shown now,
the DEFGAC scale was the missing key to what the bell casters had in mind.
12-tone system
The European 12-tone system developed as one with 7 main tones (white
keys) and 5 accidentals (black keys). The Zeng bells have 6 main tones
and 6 accidentals. As shown in the table above, there is only one complete
chromatic 12-tone scale (E4-E5 in set II). Three further octaves have
four, three, and two accidentals, respectively. Thus, a wide range of
melodic and harmonic variations was possible. It would be wrong, however,
to call the Zeng bells a 12-tone instrument, like the piano and most other
European fixed-key instruments. The accidentals had to remain accidentals,
due to their small number, and also because they were less accurately
tuned (see data tables part A).
Norm tone F
A norm tone is not essential in an orchestra. But if there is one, it
can be useful in instrument production and in performance. A norm tone
for the Zeng bells would also be a further key to the underlying tuning
system. Because each of the tones DEFGAC is repeated eight or nine times
in the 33 melody bells, there is a way to detect a possible norm tone
among them by calculating the tuning variance within each tone class and
then compare the results across tone classes. If one tone class has a
statistically significant low tuning variance, it can be considered as
the one that contains the norm tone of the system. The data show that
F is the outstanding one. The result is significant on the 0.05 level
(F-test, two-tailed, F=4.527, df1=39, df2=7). In other words, there is
a probability of less than 5 % that the exceptionally low tuning variance
in the tone class F is a chance result. The bells do not reveal directly
in which octave the norm tone was. But they clearly show that the octave
D4-D5 was the main one. It overlaps almost fully with the present international
main octave of C4-C5 (c1-c2, in traditional European terms), and also
with the main octave of Central Javanese Gamelan (CD4-CD5). This octave
encompasses the major part of the pitch range of the voices of women and
children, and it is also favored by the human auditory system (as reported
here). All indicates that the
bell casters had their norm tone in this octave, that is, close to F4
- 20 Cent relative to the modern A4 = 440 Hz (see data
tables). The frequency of this tone is close to 345 Hz.
Tuning order of the scale D-E-F-G-A-C
The second tone of the scale that was generated from F obviously was D.
It is the lowest tone in the scale, and the interval D-F is the one that
is most precisely tuned in terms of physical harmony (frequency ratio
6:5 = 316 Cent, see data tables part B). The
means of tuning data of DEGAC then show that from D two downward fifths
generated G and C and two upward fifths generated A and E. Most interesting
is that all of these four fifths are equally tempered with a size close
to 696 Cent (data tables part A) and that this
was done for the sake of improving the thirds. This finding was also confirmed
statistically by comparing the data with five tuning models.
Tuning focus on thirds
Considering the fifths and the thirds in the scale DEFGAC, it is obvious
that the tuning focus was on pure thirds, not on pure fifths. The awareness
of pure thirds may have grown with the experience of the two-tone bells
in the preceding centuries.
Conclusions
The 65 Zeng bells prove that about 2500 years ago the Chinese had fifth
generation, fifth temperament, a 12-tone system in musical practice (not
just in theory), a norm tone for an orchestral ensemble, an integration
of fifths and thirds in tuning, and a preference of pure thirds over pure
fifths. At this point in history, China was 2000 years ahead of Europe,
not only in bell casting, but also in musical acoustics. One may hope
that replicas of ancient Chinese bells that match the quality of the best
originals can soon be made, and that appropriate mallets are used to play
them. One may also hope that musicians will then make full use of the
Gamelan-like potentials of large bell ensembles, as those from the Marquis
Yi of Zeng.
Data source
The bronze division of the Shanghai Museum: Laboratory measurements of
the frequencies of Marquis Yi's set-bells of Zeng. A special issue of
Collected Essays of the Shanghai Museum, Shanghai 1982, p. 89-92 (in Chinese).
[There are earlier tone data, published in 1981. They are not complete and the methods are less well documented, but the differences between the measurements are small. The Shanghai publication contains both the frequency data and the tones in Cent derived from them. The present study only used the frequency data, because it was found that the stated Cent numbers did not correctly match the frequencies for six of the 130 tones.]
Web documents on the excavation in 1978:
Site
A, site B,
site
C
Recommended literature:
- Lothar von Falkenhausen, Suspended Music: Chime-Bells in the Culture
of Bronze Age China. Berkeley and Los Angeles: University of California
Press, 1993 [31
sample pages].
- Thomas D. Rossing, Science of Percussion Instruments, World Scientific,
Singapore, 2000, Chapter 13: "Eastern Bells".
- Shen, Sinyan, Acoustics of Ancient Chinese Bells, Scientific American,
256, 94 (1987).
Back to: auditory midbrain, First Page English, NOM Home Page